And I obsessed over the problems. Since they were in a special binder, it seemed natural to do so — this was my binder of Impossible Problems, my binder of pain.
Gradually, it became my binder of math love.
I didn’t just learn to solve them, I learned to explain them to myself. I made sure I didn’t write down my work or the answers in the binder (because then I wouldn’t really have been able to re-solve the problems), but whenever I had a question, I made sure to write it down:
Wait, how do you add fractions, again?
Why does the area for a trapezoid use the average of the top & bottom?
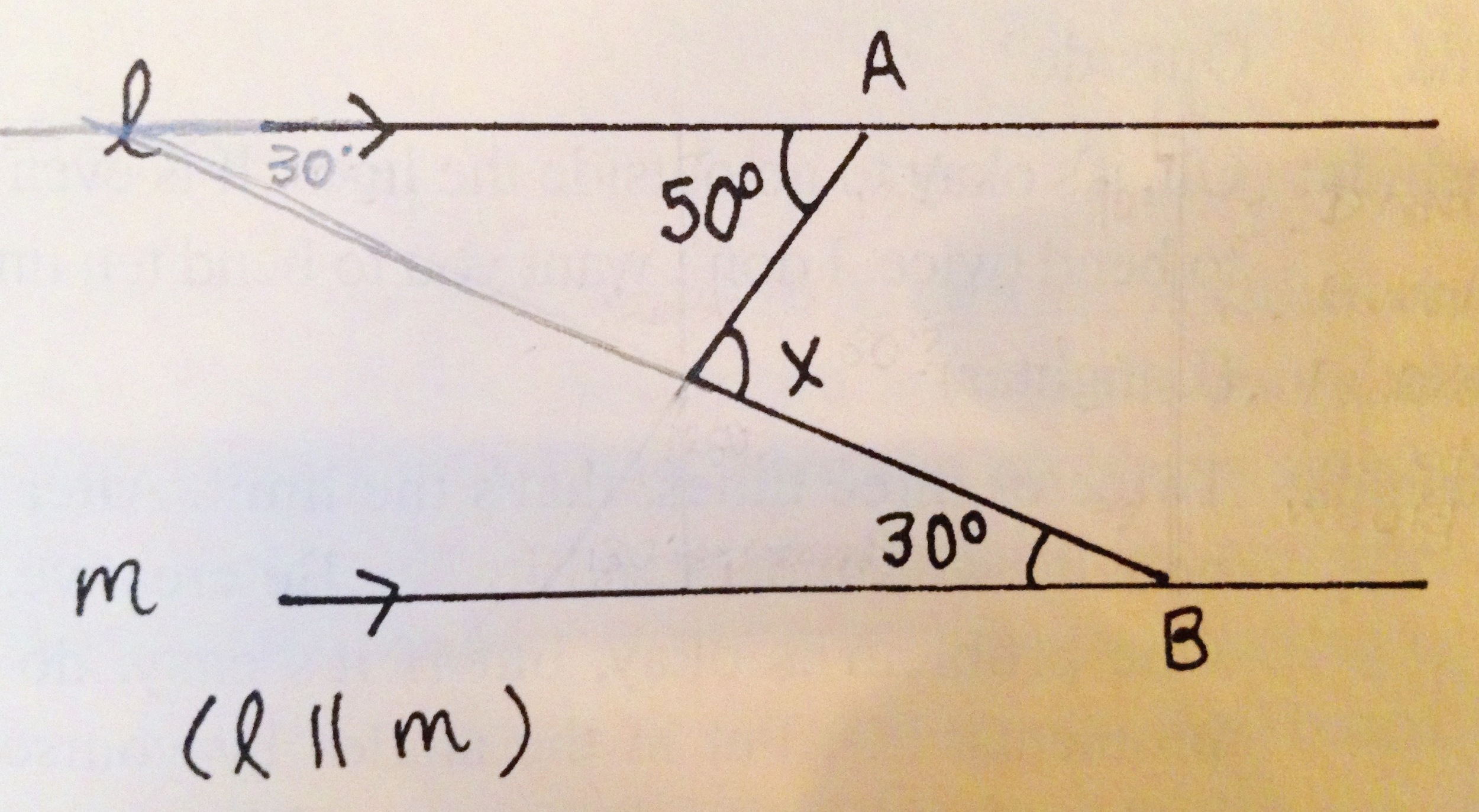
How the heck does that ugly permutation formula work?
By filling the binder with questions, and by obsessing about the answers, I learned the math so deeply I think I could have explained it to a fourth-grader.
And then, as Monday approached, I prepared to take a new half-test.
The night before the new test, I did my second oddball, I-didn’t-really-grasp-the-profundity-of-what-I-was-doing thing: I re-solved all the problems in the binder.
And was horrified when I got half of them wrong.
Remember: I had been obsessing over these problems the whole week. I had these problems down: I thought I understood them perfectly clearly.
And I got half of them wrong.
This was my first hint that human brains didn’t evolve to do GRE math. Nor did they evolve to do SAT math, or ACT math.
If I wanted to do really, really well on this test, I realized I needed to study in a fundamentally different way than twelve-plus years of schooling had prepared me to study. I needed to identify every mathematical idea I found confusing, and put it into a foolproof system that would allow me to understand it — and engrave it into my long-term memory.
Over the course of the next three months, I added problems to my binder almost religiously. And I did whatever it took to understand them — read answer explanations, pose questions, ask friends.
But all of this wouldn’t have amounted to much had I not re-solved all of them from scratch at least once each week — each and every problem I had previously entered in.
As I re-solved those problems on fresh paper, something delightful happened: I began to get them right, every time. And quickly, too! Initially I struggled with the problems, weaving back and forth inside my brain to figure out what the next step might be. But now the next steps came easily.
Before, I could only see a single step at a time — now, after re-solving the problem three or four times, I could see the whole thing at once. I could chop the problem up into tiny moves, and deal with each of those moves quickly.
And that wasn’t even the best part! About once or twice a week I would be re-solving a problem for maybe the fifth or sixth time when I’d realize that I had been an idiot. I had been solving a problem by doing a long series of steps — but if I just reconceived the problem, looked at it from a different perspective, the entire thing would be easy, could be solved in one or two moves.
Math, I realized, was simple. It was elegant. These insights were glorious — when I had them it felt like the sky was opening, and a beam of light was shining down directly on me. I could almost imagine I could hear angels singing.
And I recognized that this was why mathematicians did it — modern mathematicians, and the great mathematicians of history who had originally discovered the methods I was now uncovering myself. They were chasing the sublime high of mathematical insight.
How often, I asked myself, did I experience this in all of high school?
Maybe once or twice.
But now, studying for a standardized test — engaging in perhaps the least glamorous math learning task Western civilization has devised! — I was experiencing these epiphanies once or twice a week.
I had stumbled upon, I realized, a way of dependably building math expertise. And I was seeing it pay off: almost each week, my GRE practice test score rose. In fact, it rose quite predictably — going up about as many points as problems I had mastered in the previous week.
When I entered in 10 problems, my score went up 10–20 points.
When I entered in 20 problems, my score went up 20–40 points.
The week before I took my real test, I counted the problems I had copied into my binder — 104. And on my practice tests that week (full ones) I scored an 800 and a 790.
When I took the real GRE, I scored an 800 — a perfect score. Not bad for someone who avoided math in school.
But much better than the score was my newfound sense of myself as a mathematician. I realized that no mathematical concept was beyond me — I could understand anything, given enough time and effort. And I could enter it into a foolproof system, and, by repeatedly re-solving it, comprehend the ideas even more fully as time went by.
And I could even like it. Because to really understand something — to make sense of it inside and out, forwards and backwards — is sweet, and worth the struggle to achieve it.
Over the years since then, I’ve helped hundreds of students build their own collections of impossible problems — “magical math binders”, as one of my students has dubbed them, or “deep practice books”, as I call them.
And in a few posts to follow, I’d like to help you build and maintain your own — if you've the hankering to fall in love with math, too.